How Can Students Benefit from Multisensory Maths?

How can students benefit from multisensory maths?

When taught using hands-on multisensory ways, maths can be an easy subject to teach. By using these effective methods, students will be able grasp ideas much quicker, understand what is being taught and are able to use it in everyday life situations - as well as to achieve better results in maths class, tests and end-of-year exams.

By using step-by-step CRA methodology and a set of specific hands-on manipulatives, you can teach basic addition to advanced algebra. Students who are struggling with maths, experiencing maths anxiety, bored with maths or proclaim they hate maths can be be supported to re-engage with maths. Multisensory methods also include all 4 learning styles and support strong neurological learning pathways, with greater understanding, memory and retention of what is being taught. 

Research proven and used successfully across Australia with thousands of students, teaching maths using multisensory methods is suitable for students of all ages, grade levels and learning abilities - and is perfect for intervention, small groups, tutoring, and those with dyscalculia and dysgraphia. 

Let's start this discussion with an explanation of CRA Methodology and how to use this step-by-step process to teach maths, and any other subject. 

What is CRA methodology?

Concrete Representational Abstract (CRA) is a sequential three level strategy promoting overall conceptual understanding, procedural accuracy and fluency by employing multisensory instructional techniques when introducing the new concepts. It has been found to be highly effective in teaching math concepts in a multisensory way and has supported many students to achieve better maths results.

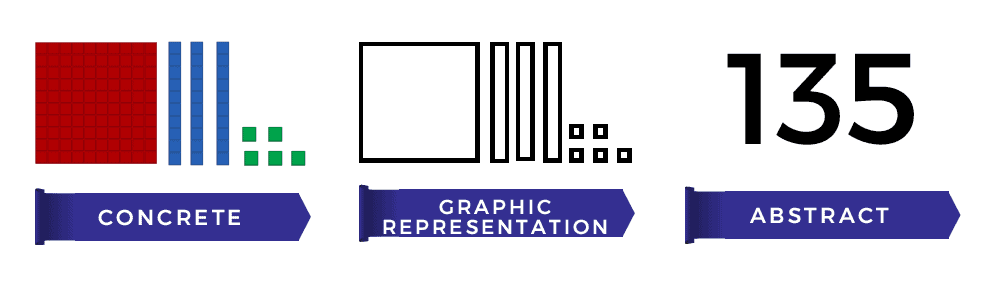
The first step is called the concrete stage.  It is known as the “doing” stage and involves physically manipulating objects to solve a math problem. During the concrete stage of instruction, three-dimensional objects are employed so students can use the manipulatives to assist while they are learning the new concept (Miller & Kaffar, 2011). The use of manipulatives increases the number of sensory inputs a student uses while learning the new concept, which improves the chances for a student to remember the procedural steps, needed to solve the problem (Witzel, 2005).

The second representational stage is the next step. It is known as the “seeing” stage and involves using images to represent objects to solve a math problem. In the representational stage of instruction, students are taught to use two-dimensional drawings (instead of the manipulatives from the concrete stage) to represent the same concepts.

The manipulations in the concrete and representational stages allow students to rationalize the conceptual mathematical procedures into logical steps and understandable definitions (Witzel, Riccomini, & Schneider, 2008).  When students encounter a difficult mathematical problem, they are able to construct pictorial representations to assist in find the solution (Witzel, 2005).

The final step in this approach is called the abstract stage.  It is known as the “symbolic” stage and involves using only numbers and symbols to solve a math problem.  CRA is a gradual systematic approach. In the abstract stage, students are taught how to translate the two-dimensional drawings into the conventional mathematics notation to solve the problem (Miller & Kaffar, 2011).

Each stage builds on to the previous stage and therefore must be taught in sequence. Sometimes, preschools and kindergartens use 3D objects to teach basic numbers such as popsicles and buttons (which is a great start!) but continuing using these basic objects isn't effective when you get into more advanced maths concepts.

What makes a multisensory approach work?

Although "multisensory maths" is becoming popular in the maths world, the concepts aren't understood or taught properly. "Multisensory" goes far beyond teaching students with coloured buttons, coloured paddle pop sticks, checkers, dominos, pizza slices or using fingers to count out a number. These are all short term "time-fillers" when teaching maths because they don't address the real issue (most students taught using a variety of filler objects when taught maths constantly struggle with simple concepts like Place Value).

Here are some examples of "hands on" items that are often used to teach maths when a) the students are in preschool or early years or b) when students are struggling with numbers, place value and maths concepts and teacher aren't given anything else to use and that don't usually work:

• coloured chips
• coloured buttons
• beans
• unifix cubes
• candy (e.g Skittles)
• popsicle sticks
• fraction blocks
• slices of pizza
• pieces of fruit

Or, teachers try and show numbers representationally using the following:

• tally marks
• dots
• circles
• pictures of objects

Why don't slices of pizza and coloured buttons work when teaching maths?

There are important reasons why using these basic objects as a "hands-on" fillers don't work. They don't allow proper learning, integration or advancement to more complex mathematical understanding. 

• They don't work when you progress to larger numbers (or you can invest in an endless supply of popsicle sticks if you have the budget but still....)
• They keep a student at the "counting phase" which is where they learn to count singular, unit numbers and don't progress to fast-counting (addition)
• It's not a long term solution - you can't use these objects in high school and advanced maths
• The most important thing is not learnt properly - student's don't understand Place Value even into high school!

What is the importance of using hands on manipulatives?

When using one set of manipulatives throughout their schooling maths curriculum and showing maths in a concrete, step-by-step way, your students will begin to understand maths as a concept that makes sense from small numbers to larger numbers and in all complex formulations. When taught correctly, they will be able to confidently achieve excellent maths results!

Here are some important benefits of using hands-on tools:

This handy complete set of manipulatives (as pictured above) is used through each lesson of the Math-U-See program from basic to advanced maths concepts, from preschool to high school and University level maths. The use of specific colours and research-proven methods to teach numbers concretely has been used successfully throughout a variety of learning environments for effective results. Modelling each mathematical concept as you teach it to your student, and providing lots of opportunities to practice is important when teaching maths.

Using multisensory tools means you take numbers off the page and make them experiential for your student. It's a much more effective way of teaching and creates strong neurological pathways for better memory, retention, integration and supports your students to achieve better maths results. 

Does a multisensory approach work for all students?

The VARK method is about acknowledging the learning styles of students and using all different styles of learning to teach a concept - visual, auditory, reading and kinaesthetic methods - and proves that students of all learning styles can benefit enormously from hands-on learning (combining both the visual + kinaesthetic learners is a huge percent!)

Here's what each learning style means for students in a learning environment:

By using multisensory methods to teach any subject, you are engaging your student through visual and kinaesthetic learning styles and covering a huge percentage of student learning pathways.

Multisensory methods are specific for students with learning differences

This method of using concrete, hands-on manipulatives has profound benefits and is especially effective for students with a variety of learning differences, including:

Looking for extra support to teach maths?

If you have the right multisensory resources and know what you're doing with them, teaching maths becomes easy and effective. By teaching maths concepts to your students in a way they understand, you'll move through maths lessons much faster, have better student engagement and get better results.

Due to high demand, we also offer Teacher-specific Trainings, so you have the reasons, methods and support to teach maths in an effective way (and how to make it work for a whole classroom full of students at all different levels!) 

You can check out the upcoming dates here:

Are you teaching your kids at home?

If you are a home-educating or homeschooling parent, there are also specific training, resources and free downloads that can help you teach your kids at home. 

We have created an Online Parent Training Course to give you the tips, tricks and simple methodology that will make teaching maths easy for any child, no matter what age or grade level. Plus, this online course is designed so you can access it anywhere you like, globally!

These skills are essential for any parent teaching their kids at home. Check out the Online Parent Course here. 

What struggles are you experiencing with your students at the moment? Let us know what IS working and what's not in the comments below

Good luck teaching maths!

Esther White

References:

Anstrom, T. (n.d.). Supporting students in mathematics through the use of manipulatives. Washington, DC: Center for Implementing Technology in Education. Retrieved April 14, 2012. 

Bender, W. (2009). Differentiating math instruction: Strategies that work for K-8 classrooms. Thousand Oaks: Corwin Press.

Sousa, D. (2008). How the brain learns mathematics. Thousand Oaks: Corwin Press.

The Access Center: Improving Outcomes for All Students K-8. (n.d.). Concrete-Representational-Abstract instructional approach. Retrieved April 14, 2012.

There are also references to the article from "Making Education Fun"